탐색(Search)

 

탐색(Search) 알고리즘 개요

탐색은 주어진 데이터에서 원하는 값을 찾는 과정을 의미합니다.

 

알고리즘	특징
완전 탐색(Brute Force)	모든 경우를 하나씩 확인
이진 탐색(Binary Search)	정렬된 배열에서 반씩 줄여가며 탐색
백트래킹(Backtracking)	재귀적으로 경우의 수를 탐색하며 불가능한 경로는 가지치기

1. 완전 탐색(Brute Force)

• 가능한 모든 경우를 직접 확인하여 답을 찾는 방법
• 효율적이지 않지만, 작은 데이터에서는 단순하고 직관적

#include <iostream>
#include <vector>
using namespace std;

int main() {
    vector<int> arr = {3, 1, 4, 1, 5, 9};
    int target = 4;
    bool found = false;

    for (int num : arr) {
        if (num == target) {
            found = true;
            break;
        }
    }
    cout << (found ? "찾았다!" : "없다!") << endl;
    return 0;
}

2. 이진 탐색(Binary Search)

• 정렬된 배열에서 탐색을 빠르게 수행하는 알고리즘
• 중앙값을 기준으로 절반씩 줄여가며 탐색

#include <iostream>
#include <vector>
using namespace std;

bool binarySearch(vector<int>& arr, int target) {
    int left = 0;
    int right = arr.size() - 1;

    while (left <= right) {
        int mid = left + (right - left) / 2;

        if (arr[mid] == target) {
            return true;
        }
        else if (arr[mid] < target) {
            left = mid + 1;
        }
        else {
            right = mid - 1;
        }
    }

    return false;
}

bool binarySearchRecursive(vector<int>& arr, int left, int right, int target) {
    if (left > right) {
        return false;
    }

    int mid = left + (right - left) / 2;

    if (arr[mid] == target) {
        return true;
    }

    if (arr[mid] < target) {
        return binarySearchRecursive(arr, mid + 1, right, target);
    }

    return binarySearchRecursive(arr, left, mid - 1, target);
}


int main() {
    vector<int> arr = { 1, 3, 5, 7, 9, 11 }; // 정렬된 배열

    cout << (binarySearch(arr, 5) ? "찾았다!" : "없다!") << endl;
    cout << (binarySearchRecursive(arr, 0, arr.size() - 1, 5) ? "찾았다!" : "없다!") << endl;

    return 0;
}

3. 백트래킹(Backtracking)

• 모든 경우의 수를 탐색하지만, 불가능한 경로는 가지치기
• 주로 조합, 순열, 그래프 탐색 등에 사용

#include <iostream>
#include <vector>
using namespace std;

void backtrack(vector<int>& nums, vector<bool>& used, vector<int>& current) {
    if (current.size() == nums.size()) {

        for (int num : current) {
            cout << num << " ";
        }

        cout << endl;

        return;
    }

    for (int i = 0; i < nums.size(); i++) {
        if (!used[i]) {
            used[i] = true;
            current.push_back(nums[i]);
            backtrack(nums, used, current);
            current.pop_back();
            used[i] = false;
        }
    }
}

int main() {
    vector<int> nums = { 1, 2, 3 };
    vector<bool> used(nums.size(), false);
    vector<int> current;

    backtrack(nums, used, current);

    return 0;
}

그래프 탐색(Graph Traversal)

그래프 탐색은 노드(Node)와 간선(Edge)으로 이루어진 그래프에서 특정 노드들을 방문하는 과정입니다.

 

• V : 정점(Vertex)의 개수
• E : 간선(Edge)의 개수

 

알고리즘	탐색 방식	사용 구조	시간 복잡도
DFS(깊이 우선 탐색)	깊이(하위 노드)부터 탐색	재귀(스택)	O(V+E)
BFS(너비 우선 탐색)	가까운 노드부터 탐색	큐	O(V+E)

 

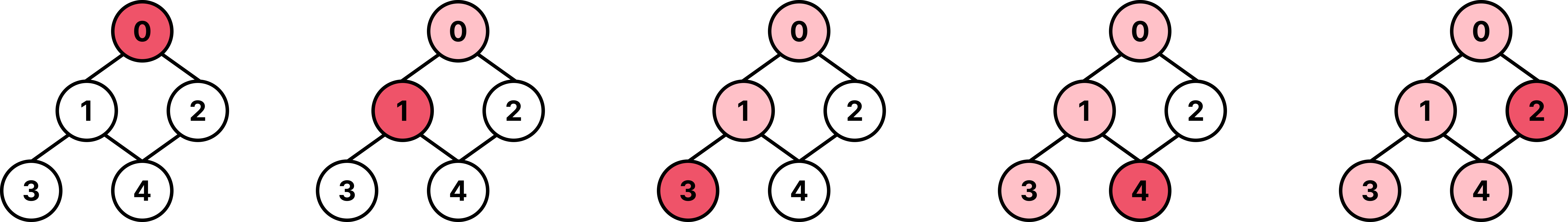
1. DFS(Depth-First Search, 깊이 우선 탐색)

• 한 방향으로 최대한 깊이 탐색 후, 다시 돌아와 다른 경로 탐색
• 스택이나 재귀를 이용해서 구현 가능

 

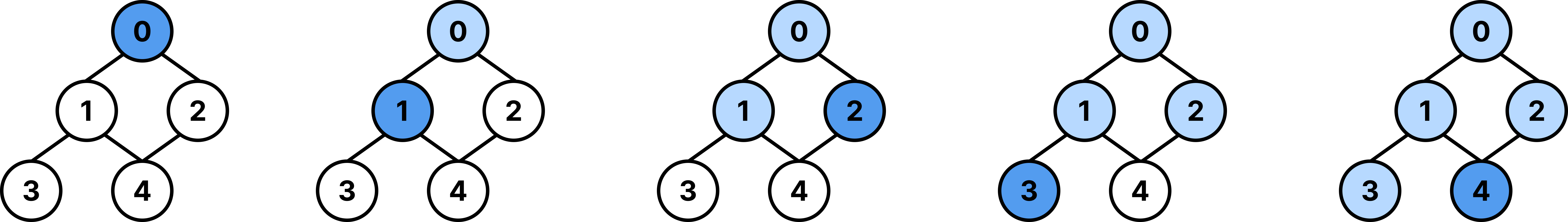
탐색 흐름

• 0 방문 ➡️ 1 방문 ➡️ 3 방문 (더 깊이 갈 곳 없음, 1로 되돌아감)
• 4 방문 (더 깊이 갈 곳 없음, 1로 되돌아감 ➡️ 더 깊이 갈 곳 없음, 0으로 되돌아감)
• 2 방문 ➡️ 4는 이미 방문됨 (종료)

 

#include <iostream>
#include <vector>
using namespace std;

vector<vector<int>> graph;
vector<bool> visited;

void dfs(int node) {
    visited[node] = true;
    cout << node << " ";

    for (int next : graph[node]) {
        if (!visited[next]) {
            dfs(next);
        }
    }
}

int main() {
    int V = 5; // 노드 개수
    graph.resize(V);
    visited.resize(V, false);

    // 그래프 생성 (0-1, 0-2, 1-3, 1-4, 2-4)
    graph[0] = { 1, 2 };
    graph[1] = { 0, 3, 4 };
    graph[2] = { 0, 4 };
    graph[3] = { 1 };
    graph[4] = { 1, 2 };

    cout << "DFS 탐색 순서: ";
    dfs(0); // 0 1 3 4 2
    return 0;
}

2. BFS(Breadth-First Search, 너비 우선 탐색)

• 가까운 노드부터 탐색
• 최단 경로 문제에 자주 사용됨

 

탐색 흐름

• 0 방문 ➡️ 1, 2 큐에 추가 
• 큐에 있는 1 방문 ➡️ 3, 4 큐에 추가
• 큐에 있는 2 방문 ➡️ 4는 이미 추가됨
• 큐에 있는 3 방문 (더 탐색할 곳 없음)
• 큐에 있는 4 방문 (더 탐색할 곳 없음)

 

#include <iostream>
#include <vector>
#include <queue>
using namespace std;

vector<vector<int>> graph;
vector<bool> visited;

void bfs(int start) {
    queue<int> q;
    q.push(start);
    visited[start] = true;

    while (!q.empty()) {
        int node = q.front();
        q.pop();
        cout << node << " ";

        for (int next : graph[node]) {
            if (!visited[next]) {
                visited[next] = true;
                q.push(next);
            }
        }
    }
}

int main() {
    int V = 5; // 노드 개수
    graph.resize(V);
    visited.resize(V, false);

    // 그래프 생성 (0-1, 0-2, 1-3, 1-4, 2-4)
    graph[0] = { 1, 2 };
    graph[1] = { 0, 3, 4 };
    graph[2] = { 0, 4 };
    graph[3] = { 1 };
    graph[4] = { 1, 2 };

    cout << "BFS 탐색 순서: ";
    bfs(0); // 0 1 2 3 4
    return 0;
}